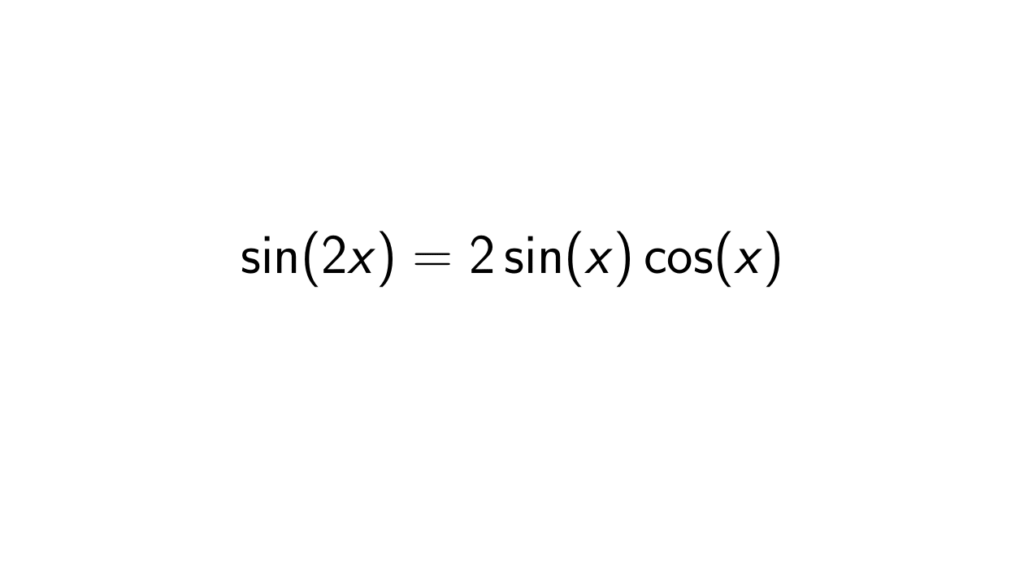Cosx 2 Sinx 2
Cosx 2 Sinx 2 - Since both terms are perfect squares, factor using the difference of squares formula, where and. X^{\msquare} \log_{\msquare} \sqrt{\square} \nthroot[\msquare]{\square} \le \ge \frac{\msquare}{\msquare} \cdot \div: Compute answers using wolfram's breakthrough technology & knowledgebase, relied on by millions of students & professionals. Since both terms are perfect squares, factor using the difference of squares formula, where and.
Since both terms are perfect squares, factor using the difference of squares formula, where and. Since both terms are perfect squares, factor using the difference of squares formula, where and. X^{\msquare} \log_{\msquare} \sqrt{\square} \nthroot[\msquare]{\square} \le \ge \frac{\msquare}{\msquare} \cdot \div: Compute answers using wolfram's breakthrough technology & knowledgebase, relied on by millions of students & professionals.
Since both terms are perfect squares, factor using the difference of squares formula, where and. Since both terms are perfect squares, factor using the difference of squares formula, where and. X^{\msquare} \log_{\msquare} \sqrt{\square} \nthroot[\msquare]{\square} \le \ge \frac{\msquare}{\msquare} \cdot \div: Compute answers using wolfram's breakthrough technology & knowledgebase, relied on by millions of students & professionals.
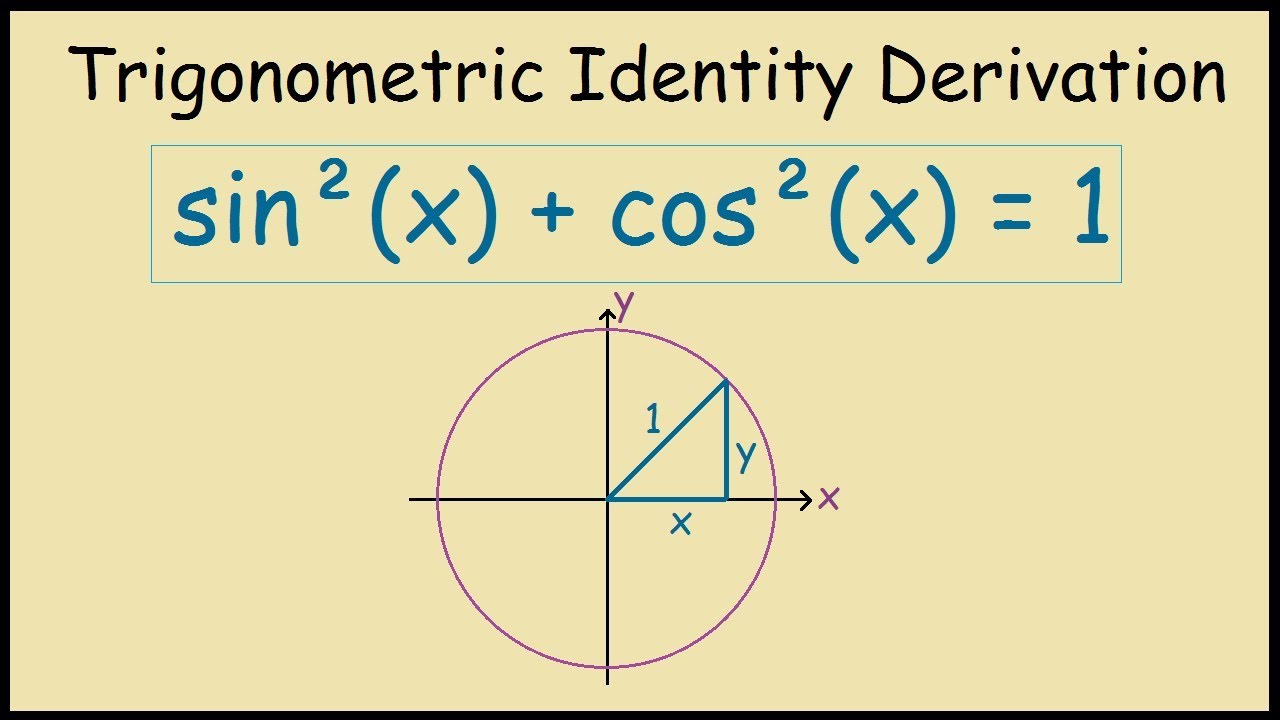
sin^2(x) + cos^2(x) = 1 Trig Identity Graphical Proof YouTube
Compute answers using wolfram's breakthrough technology & knowledgebase, relied on by millions of students & professionals. Since both terms are perfect squares, factor using the difference of squares formula, where and. X^{\msquare} \log_{\msquare} \sqrt{\square} \nthroot[\msquare]{\square} \le \ge \frac{\msquare}{\msquare} \cdot \div: Since both terms are perfect squares, factor using the difference of squares formula, where and.
Ex 7.3, 20 Integrate cos 2x / (cos x + sin x)^2 NCERT Maths
Compute answers using wolfram's breakthrough technology & knowledgebase, relied on by millions of students & professionals. Since both terms are perfect squares, factor using the difference of squares formula, where and. Since both terms are perfect squares, factor using the difference of squares formula, where and. X^{\msquare} \log_{\msquare} \sqrt{\square} \nthroot[\msquare]{\square} \le \ge \frac{\msquare}{\msquare} \cdot \div:
Prove (sinx+cosx)^2=sin2x+1 YouTube
Since both terms are perfect squares, factor using the difference of squares formula, where and. Since both terms are perfect squares, factor using the difference of squares formula, where and. Compute answers using wolfram's breakthrough technology & knowledgebase, relied on by millions of students & professionals. X^{\msquare} \log_{\msquare} \sqrt{\square} \nthroot[\msquare]{\square} \le \ge \frac{\msquare}{\msquare} \cdot \div:
Prove that sin(2x) = 2sin(x)cos(x) Epsilonify
Compute answers using wolfram's breakthrough technology & knowledgebase, relied on by millions of students & professionals. X^{\msquare} \log_{\msquare} \sqrt{\square} \nthroot[\msquare]{\square} \le \ge \frac{\msquare}{\msquare} \cdot \div: Since both terms are perfect squares, factor using the difference of squares formula, where and. Since both terms are perfect squares, factor using the difference of squares formula, where and.
Integral of (sinx + cosx)^2 YouTube
Since both terms are perfect squares, factor using the difference of squares formula, where and. X^{\msquare} \log_{\msquare} \sqrt{\square} \nthroot[\msquare]{\square} \le \ge \frac{\msquare}{\msquare} \cdot \div: Since both terms are perfect squares, factor using the difference of squares formula, where and. Compute answers using wolfram's breakthrough technology & knowledgebase, relied on by millions of students & professionals.
Prove that(sin xcos x)^2 =1sin 2x
Since both terms are perfect squares, factor using the difference of squares formula, where and. Since both terms are perfect squares, factor using the difference of squares formula, where and. X^{\msquare} \log_{\msquare} \sqrt{\square} \nthroot[\msquare]{\square} \le \ge \frac{\msquare}{\msquare} \cdot \div: Compute answers using wolfram's breakthrough technology & knowledgebase, relied on by millions of students & professionals.
Pembuktian cos2x=cos^2xsin^2x dan sin 2x=2sinxcosx Trigonometry
X^{\msquare} \log_{\msquare} \sqrt{\square} \nthroot[\msquare]{\square} \le \ge \frac{\msquare}{\msquare} \cdot \div: Since both terms are perfect squares, factor using the difference of squares formula, where and. Compute answers using wolfram's breakthrough technology & knowledgebase, relied on by millions of students & professionals. Since both terms are perfect squares, factor using the difference of squares formula, where and.
Proof of cos2x=(cosx)^2(sinx)^2=2(cosx)^2 1=12(sinx)^2 YouTube
Since both terms are perfect squares, factor using the difference of squares formula, where and. X^{\msquare} \log_{\msquare} \sqrt{\square} \nthroot[\msquare]{\square} \le \ge \frac{\msquare}{\msquare} \cdot \div: Since both terms are perfect squares, factor using the difference of squares formula, where and. Compute answers using wolfram's breakthrough technology & knowledgebase, relied on by millions of students & professionals.
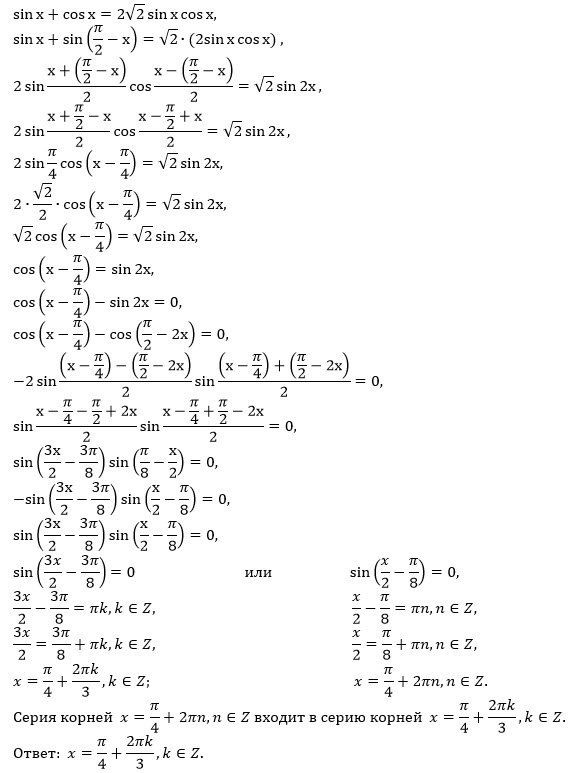
sinx+cosx = 2sqrt(2)sinx*cosx
Since both terms are perfect squares, factor using the difference of squares formula, where and. Compute answers using wolfram's breakthrough technology & knowledgebase, relied on by millions of students & professionals. X^{\msquare} \log_{\msquare} \sqrt{\square} \nthroot[\msquare]{\square} \le \ge \frac{\msquare}{\msquare} \cdot \div: Since both terms are perfect squares, factor using the difference of squares formula, where and.
find value of sinx/2 , cosx/2 ,tanx/2if..1. cosx = 1/3 x is in third
Compute answers using wolfram's breakthrough technology & knowledgebase, relied on by millions of students & professionals. X^{\msquare} \log_{\msquare} \sqrt{\square} \nthroot[\msquare]{\square} \le \ge \frac{\msquare}{\msquare} \cdot \div: Since both terms are perfect squares, factor using the difference of squares formula, where and. Since both terms are perfect squares, factor using the difference of squares formula, where and.
Since Both Terms Are Perfect Squares, Factor Using The Difference Of Squares Formula, Where And.
Since both terms are perfect squares, factor using the difference of squares formula, where and. X^{\msquare} \log_{\msquare} \sqrt{\square} \nthroot[\msquare]{\square} \le \ge \frac{\msquare}{\msquare} \cdot \div: Compute answers using wolfram's breakthrough technology & knowledgebase, relied on by millions of students & professionals.