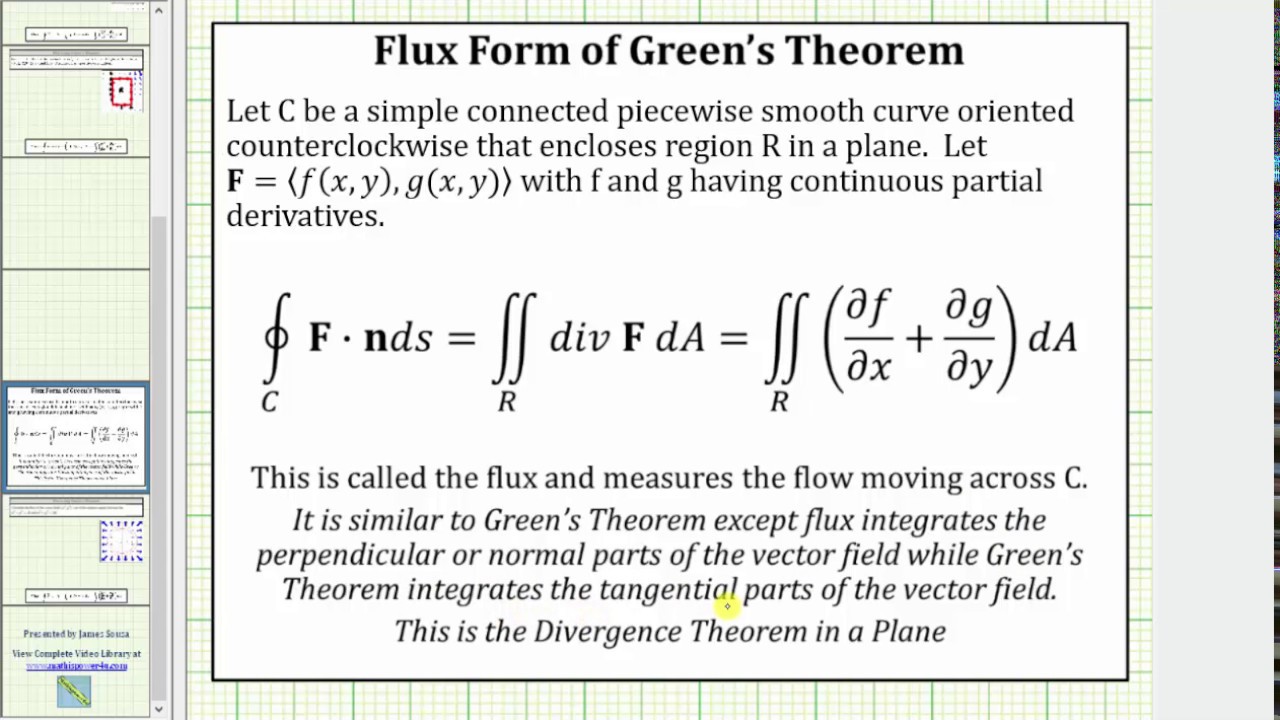
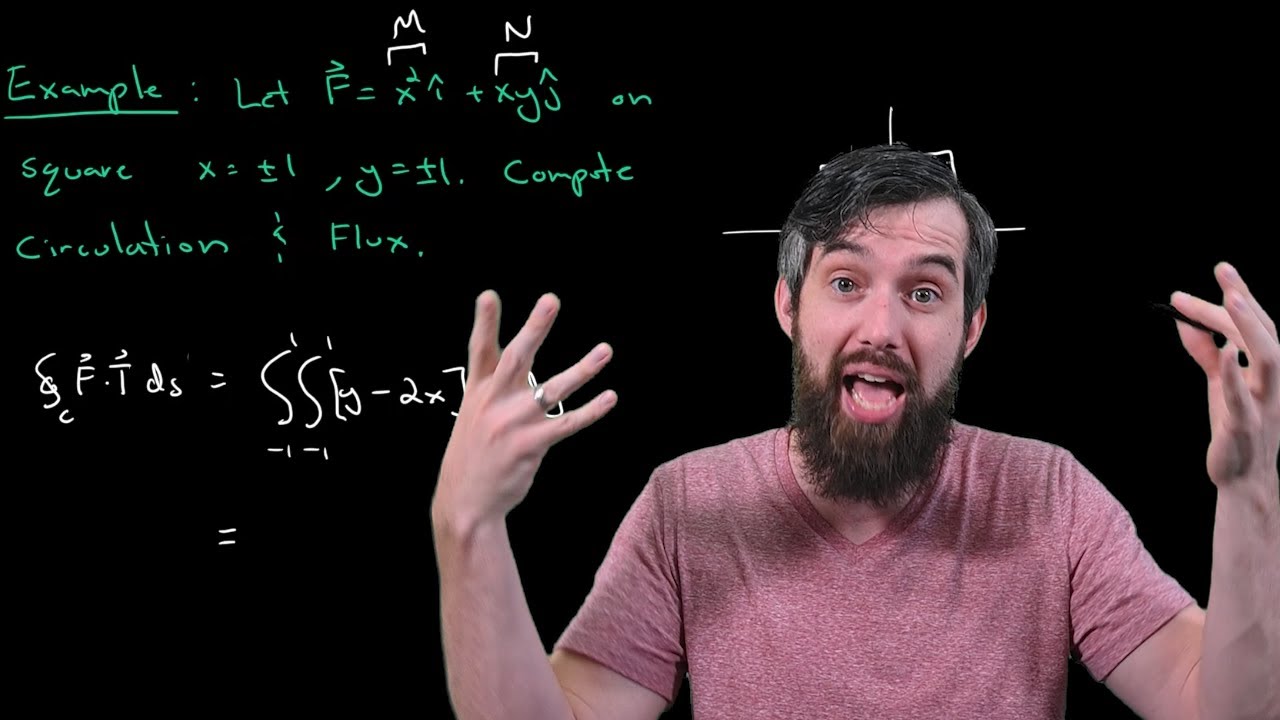Flux Form Of Green S Theorem
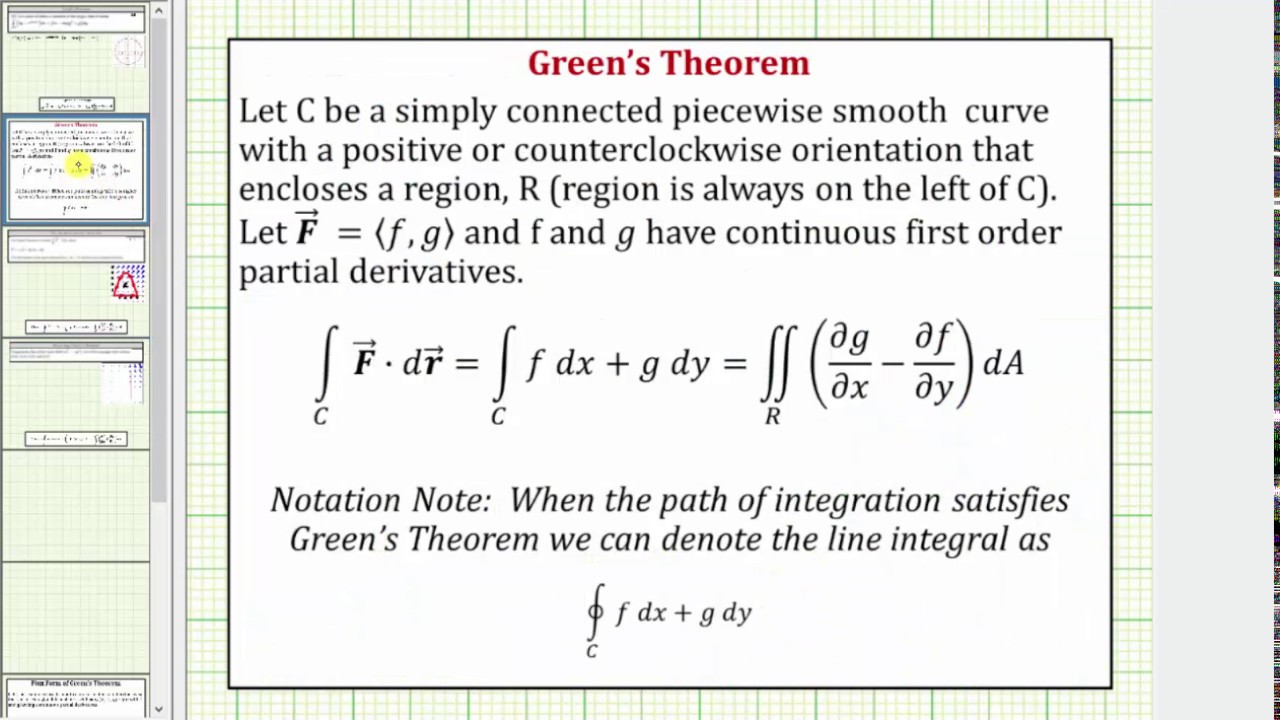
Flux Form Of Green S Theorem - The flux of a fluid. In a similar way, the flux form of green’s theorem follows from the circulation form: The flux form of green’s theorem relates a double integral over region \(d\) to the flux across boundary \(c\). We substitute l(f) in place of f in equation (2) and use the. The flux form of green’s theorem relates a double integral over region [latex]d[/latex] to the flux across curve [latex]c[/latex]. Green's theorem can be used to find the area of a 2d shape. The integral we would normally use to calculate the area is just \iint_r 1\,da ∬ r1da.
The flux form of green’s theorem relates a double integral over region \(d\) to the flux across boundary \(c\). We substitute l(f) in place of f in equation (2) and use the. Green's theorem can be used to find the area of a 2d shape. The integral we would normally use to calculate the area is just \iint_r 1\,da ∬ r1da. In a similar way, the flux form of green’s theorem follows from the circulation form: The flux form of green’s theorem relates a double integral over region [latex]d[/latex] to the flux across curve [latex]c[/latex]. The flux of a fluid.
Green's theorem can be used to find the area of a 2d shape. We substitute l(f) in place of f in equation (2) and use the. In a similar way, the flux form of green’s theorem follows from the circulation form: The flux form of green’s theorem relates a double integral over region \(d\) to the flux across boundary \(c\). The integral we would normally use to calculate the area is just \iint_r 1\,da ∬ r1da. The flux form of green’s theorem relates a double integral over region [latex]d[/latex] to the flux across curve [latex]c[/latex]. The flux of a fluid.
Determine the Flux of a 2D Vector Field Using Green's Theorem (Parabola
The integral we would normally use to calculate the area is just \iint_r 1\,da ∬ r1da. The flux form of green’s theorem relates a double integral over region [latex]d[/latex] to the flux across curve [latex]c[/latex]. The flux of a fluid. In a similar way, the flux form of green’s theorem follows from the circulation form: We substitute l(f) in place.
Flux Form of Green's Theorem Vector Calculus YouTube
Green's theorem can be used to find the area of a 2d shape. The flux form of green’s theorem relates a double integral over region [latex]d[/latex] to the flux across curve [latex]c[/latex]. The integral we would normally use to calculate the area is just \iint_r 1\,da ∬ r1da. In a similar way, the flux form of green’s theorem follows from.
Green's Theorem Flux Form YouTube
The integral we would normally use to calculate the area is just \iint_r 1\,da ∬ r1da. The flux form of green’s theorem relates a double integral over region \(d\) to the flux across boundary \(c\). Green's theorem can be used to find the area of a 2d shape. In a similar way, the flux form of green’s theorem follows from.
Flux Form of Green's Theorem YouTube
Green's theorem can be used to find the area of a 2d shape. We substitute l(f) in place of f in equation (2) and use the. In a similar way, the flux form of green’s theorem follows from the circulation form: The flux form of green’s theorem relates a double integral over region \(d\) to the flux across boundary \(c\)..
Example Using Green's Theorem to Compute Circulation & Flux // Vector
The flux of a fluid. The flux form of green’s theorem relates a double integral over region [latex]d[/latex] to the flux across curve [latex]c[/latex]. Green's theorem can be used to find the area of a 2d shape. The flux form of green’s theorem relates a double integral over region \(d\) to the flux across boundary \(c\). In a similar way,.
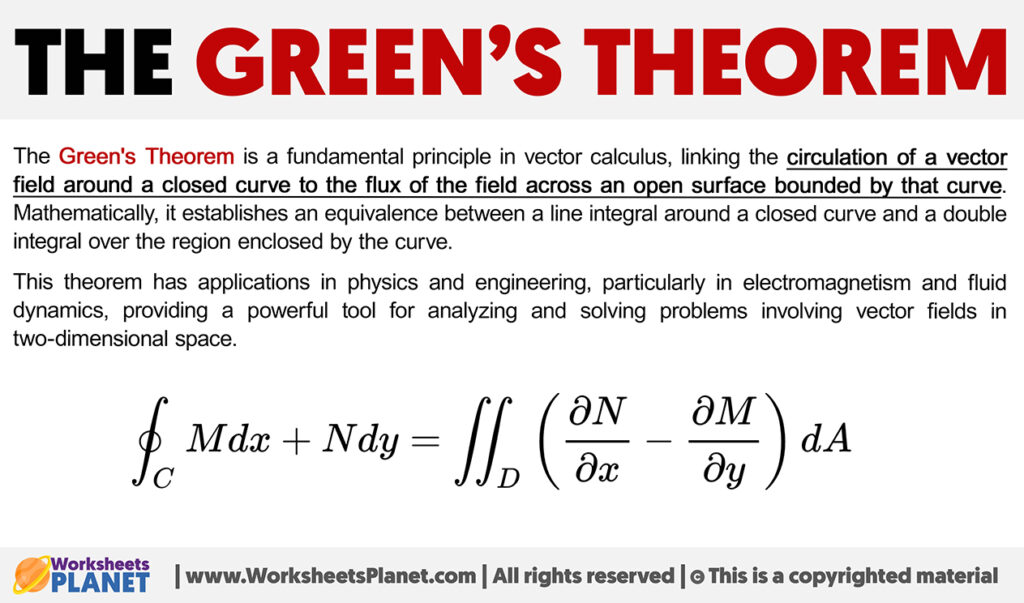
The Green's Theorem Formula + Definition
The flux of a fluid. The flux form of green’s theorem relates a double integral over region [latex]d[/latex] to the flux across curve [latex]c[/latex]. The integral we would normally use to calculate the area is just \iint_r 1\,da ∬ r1da. In a similar way, the flux form of green’s theorem follows from the circulation form: We substitute l(f) in place.
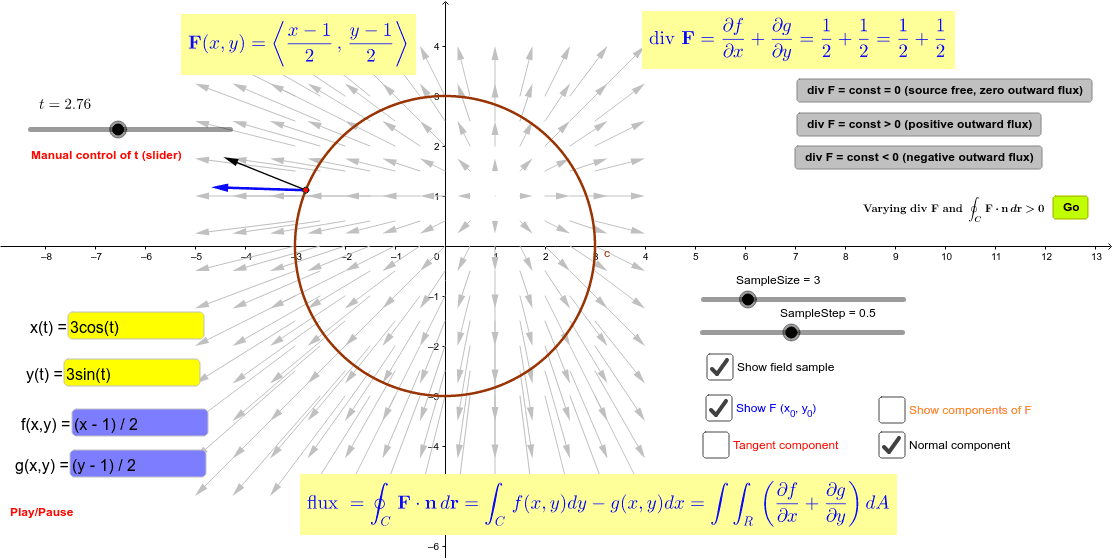
Illustration of the flux form of the Green's Theorem GeoGebra
The flux form of green’s theorem relates a double integral over region \(d\) to the flux across boundary \(c\). In a similar way, the flux form of green’s theorem follows from the circulation form: The flux of a fluid. Green's theorem can be used to find the area of a 2d shape. The flux form of green’s theorem relates a.
Determine the Flux of a 2D Vector Field Using Green's Theorem
In a similar way, the flux form of green’s theorem follows from the circulation form: We substitute l(f) in place of f in equation (2) and use the. Green's theorem can be used to find the area of a 2d shape. The integral we would normally use to calculate the area is just \iint_r 1\,da ∬ r1da. The flux form.
Determine the Flux of a 2D Vector Field Using Green's Theorem (Hole
In a similar way, the flux form of green’s theorem follows from the circulation form: The flux of a fluid. The integral we would normally use to calculate the area is just \iint_r 1\,da ∬ r1da. We substitute l(f) in place of f in equation (2) and use the. The flux form of green’s theorem relates a double integral over.
Multivariable Calculus Green's Theorem YouTube
The integral we would normally use to calculate the area is just \iint_r 1\,da ∬ r1da. We substitute l(f) in place of f in equation (2) and use the. The flux form of green’s theorem relates a double integral over region \(d\) to the flux across boundary \(c\). In a similar way, the flux form of green’s theorem follows from.
The Integral We Would Normally Use To Calculate The Area Is Just \Iint_R 1\,Da ∬ R1Da.
The flux form of green’s theorem relates a double integral over region [latex]d[/latex] to the flux across curve [latex]c[/latex]. The flux of a fluid. We substitute l(f) in place of f in equation (2) and use the. The flux form of green’s theorem relates a double integral over region \(d\) to the flux across boundary \(c\).
Green's Theorem Can Be Used To Find The Area Of A 2D Shape.
In a similar way, the flux form of green’s theorem follows from the circulation form: