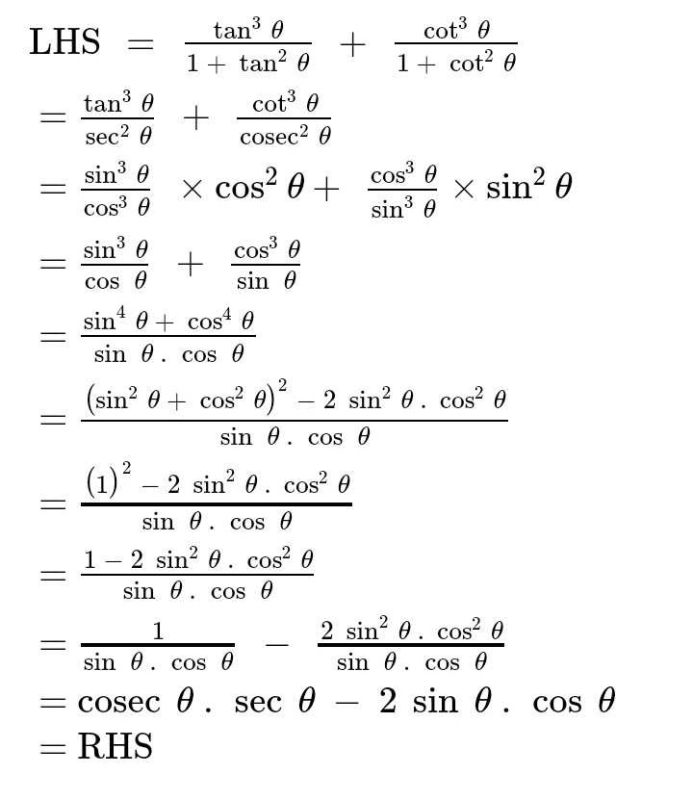Tan Theta To Cos Theta

Tan Theta To Cos Theta - Then, write the equation in a standard form, and isolate the. Cos (θ) = adjacent / hypotenuse. ∙ xsin2θ +cos2θ = 1. ∙ xtanθ = sinθ cosθ. To solve a trigonometric simplify the equation using trigonometric identities. Express tan θ in terms of cos θ? ⇒ sinθ = ± √1 −. Given sinθ = 116 and secθ>0 , how do you find cosθ,tanθ ? \displaystyle {\cos {\theta}}=\frac {\sqrt { {85}}} { {11}} and \displaystyle {\tan. Sin (θ) = opposite / hypotenuse.

\displaystyle {\cos {\theta}}=\frac {\sqrt { {85}}} { {11}} and \displaystyle {\tan. ∙ xsin2θ +cos2θ = 1. ⇒ sinθ = ± √1 −. In trigonometry formulas, we will learn all the basic formulas based on trigonometry ratios (sin,cos, tan) and identities as per class. Given sinθ = 116 and secθ>0 , how do you find cosθ,tanθ ? Cos (θ) = adjacent / hypotenuse. Then, write the equation in a standard form, and isolate the. For a right triangle with an angle θ : Express tan θ in terms of cos θ? To solve a trigonometric simplify the equation using trigonometric identities.

⇒ sinθ = ± √1 −. Cos (θ) = adjacent / hypotenuse. For a right triangle with an angle θ : Then, write the equation in a standard form, and isolate the. In trigonometry formulas, we will learn all the basic formulas based on trigonometry ratios (sin,cos, tan) and identities as per class. Express tan θ in terms of cos θ? ∙ xtanθ = sinθ cosθ. Rewrite tan(θ)cos(θ) tan (θ) cos (θ) in terms of sines and cosines. Sin (θ) = opposite / hypotenuse. To solve a trigonometric simplify the equation using trigonometric identities.

$=\frac{\sin \theta(1+\cos \theta)+\tan \theta(1\cos \theta)}{(1\cos \th..$

=\frac{\sin \theta(1+\cos \theta)+\tan \theta(1\cos \theta)}{(1\cos \th..

To solve a trigonometric simplify the equation using trigonometric identities. Given sinθ = 116 and secθ>0 , how do you find cosθ,tanθ ? Sin (θ) = opposite / hypotenuse. Express tan θ in terms of cos θ? Cos (θ) = adjacent / hypotenuse.

$\4.Provethat\frac{\tan \theta}{1\tan \theta}\frac{\cot \theta}{1\cot$

\4.Provethat\frac{\tan \theta}{1\tan \theta}\frac{\cot \theta}{1\cot

Rewrite tan(θ)cos(θ) tan (θ) cos (θ) in terms of sines and cosines. ∙ xtanθ = sinθ cosθ. For a right triangle with an angle θ : ⇒ sinθ = ± √1 −. \displaystyle {\cos {\theta}}=\frac {\sqrt { {85}}} { {11}} and \displaystyle {\tan.

Tan thetacot theta =0 then find the value of sin theta +cos theta

⇒ sinθ = ± √1 −. To solve a trigonometric simplify the equation using trigonometric identities. Express tan θ in terms of cos θ? ∙ xtanθ = sinθ cosθ. Rewrite tan(θ)cos(θ) tan (θ) cos (θ) in terms of sines and cosines.

Tan Theta Formula, Definition , Solved Examples

Then, write the equation in a standard form, and isolate the. Given sinθ = 116 and secθ>0 , how do you find cosθ,tanθ ? In trigonometry formulas, we will learn all the basic formulas based on trigonometry ratios (sin,cos, tan) and identities as per class. ⇒ sinθ = ± √1 −. To solve a trigonometric simplify the equation using trigonometric.

選択した画像 (tan^2 theta)/((sec theta1)^2)=(1 cos theta)/(1cos theta) 274439

Then, write the equation in a standard form, and isolate the. To solve a trigonometric simplify the equation using trigonometric identities. ∙ xtanθ = sinθ cosθ. Express tan θ in terms of cos θ? For a right triangle with an angle θ :

tan theta+sec theta1/tan thetasec theta+1=1+sin theta/cos theta

Given sinθ = 116 and secθ>0 , how do you find cosθ,tanθ ? Cos (θ) = adjacent / hypotenuse. In trigonometry formulas, we will learn all the basic formulas based on trigonometry ratios (sin,cos, tan) and identities as per class. ∙ xsin2θ +cos2θ = 1. Sin (θ) = opposite / hypotenuse.

画像 prove that tan^2 theta/1 tan^2 theta 298081Prove that cos 2 theta

Cos (θ) = adjacent / hypotenuse. In trigonometry formulas, we will learn all the basic formulas based on trigonometry ratios (sin,cos, tan) and identities as per class. For a right triangle with an angle θ : To solve a trigonometric simplify the equation using trigonometric identities. Rewrite tan(θ)cos(θ) tan (θ) cos (θ) in terms of sines and cosines.

tan theta/1cot theta + cot theta/1tan theta= 1+ sec theta cosec theta

Given sinθ = 116 and secθ>0 , how do you find cosθ,tanθ ? Express tan θ in terms of cos θ? ⇒ sinθ = ± √1 −. \displaystyle {\cos {\theta}}=\frac {\sqrt { {85}}} { {11}} and \displaystyle {\tan. Then, write the equation in a standard form, and isolate the.

Prove that ` (sin theta "cosec" theta )(cos theta sec theta )=(1

Sin (θ) = opposite / hypotenuse. To solve a trigonometric simplify the equation using trigonometric identities. Rewrite tan(θ)cos(θ) tan (θ) cos (θ) in terms of sines and cosines. Express tan θ in terms of cos θ? ⇒ sinθ = ± √1 −.

Find the exact expressions for sin theta, cos theta, and tan theta. sin

⇒ sinθ = ± √1 −. In trigonometry formulas, we will learn all the basic formulas based on trigonometry ratios (sin,cos, tan) and identities as per class. Cos (θ) = adjacent / hypotenuse. Express tan θ in terms of cos θ? Sin (θ) = opposite / hypotenuse.

Sin (Θ) = Opposite / Hypotenuse.

For a right triangle with an angle θ : Then, write the equation in a standard form, and isolate the. ∙ xtanθ = sinθ cosθ. ∙ xsin2θ +cos2θ = 1.

Express Tan Θ In Terms Of Cos Θ?

⇒ sinθ = ± √1 −. Rewrite tan(θ)cos(θ) tan (θ) cos (θ) in terms of sines and cosines. Given sinθ = 116 and secθ>0 , how do you find cosθ,tanθ ? To solve a trigonometric simplify the equation using trigonometric identities.