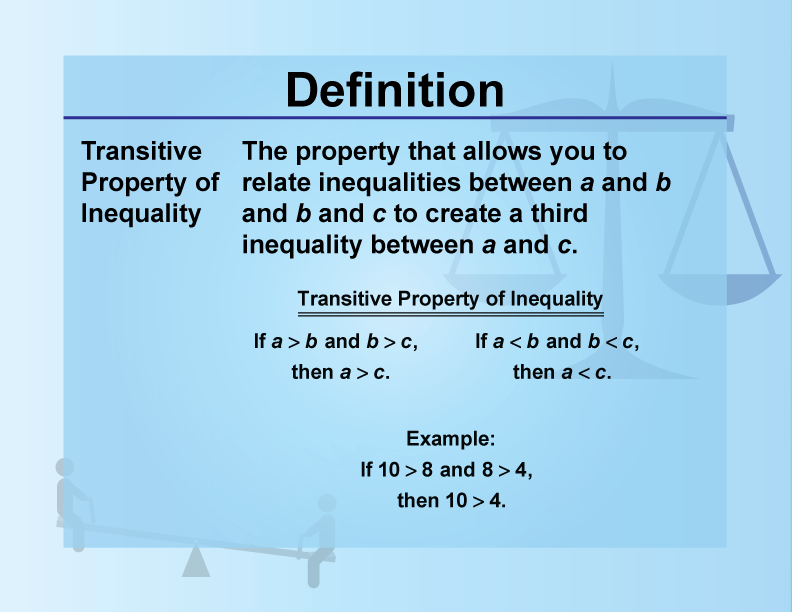
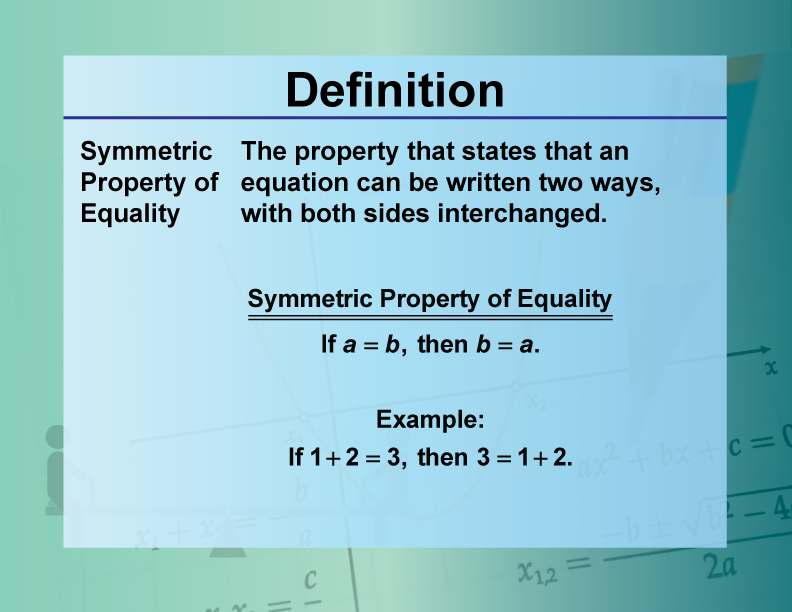
Transitive Definition Math
Transitive Definition Math - It states that if two values are equal, and either of those two values. What is the transitive property in maths? Visit byju’s to learn the statement of the transitive property, transitive property of equality and. A transitive relation is a fundamental concept in mathematics, specifically in the field of set theory and relations. The transitive property is also known as the transitive property of equality. Transitive relations are binary relations in set theory that are defined on a set a such that if a is related to b and b is related to c, then element a.
The transitive property is also known as the transitive property of equality. It states that if two values are equal, and either of those two values. Transitive relations are binary relations in set theory that are defined on a set a such that if a is related to b and b is related to c, then element a. Visit byju’s to learn the statement of the transitive property, transitive property of equality and. A transitive relation is a fundamental concept in mathematics, specifically in the field of set theory and relations. What is the transitive property in maths?
The transitive property is also known as the transitive property of equality. A transitive relation is a fundamental concept in mathematics, specifically in the field of set theory and relations. What is the transitive property in maths? It states that if two values are equal, and either of those two values. Transitive relations are binary relations in set theory that are defined on a set a such that if a is related to b and b is related to c, then element a. Visit byju’s to learn the statement of the transitive property, transitive property of equality and.
Transitive Property
What is the transitive property in maths? Visit byju’s to learn the statement of the transitive property, transitive property of equality and. The transitive property is also known as the transitive property of equality. A transitive relation is a fundamental concept in mathematics, specifically in the field of set theory and relations. Transitive relations are binary relations in set theory.
Transitive Property
What is the transitive property in maths? The transitive property is also known as the transitive property of equality. A transitive relation is a fundamental concept in mathematics, specifically in the field of set theory and relations. Transitive relations are binary relations in set theory that are defined on a set a such that if a is related to b.
Transitive Property Definition Math
A transitive relation is a fundamental concept in mathematics, specifically in the field of set theory and relations. It states that if two values are equal, and either of those two values. The transitive property is also known as the transitive property of equality. Transitive relations are binary relations in set theory that are defined on a set a such.
Transitive Property Of Multiplication propertyvb
It states that if two values are equal, and either of those two values. Transitive relations are binary relations in set theory that are defined on a set a such that if a is related to b and b is related to c, then element a. A transitive relation is a fundamental concept in mathematics, specifically in the field of.
Transitive Property of Equality Definition & Example Video & Lesson
The transitive property is also known as the transitive property of equality. A transitive relation is a fundamental concept in mathematics, specifically in the field of set theory and relations. Transitive relations are binary relations in set theory that are defined on a set a such that if a is related to b and b is related to c, then.
DefinitionInequality ConceptsTransitive Property Media4Math
A transitive relation is a fundamental concept in mathematics, specifically in the field of set theory and relations. Transitive relations are binary relations in set theory that are defined on a set a such that if a is related to b and b is related to c, then element a. It states that if two values are equal, and either.
Transitive Property of Equality YouTube
The transitive property is also known as the transitive property of equality. It states that if two values are equal, and either of those two values. What is the transitive property in maths? Transitive relations are binary relations in set theory that are defined on a set a such that if a is related to b and b is related.
Transitive Verb Definition, Types of Transitive Verbs with Useful
It states that if two values are equal, and either of those two values. A transitive relation is a fundamental concept in mathematics, specifically in the field of set theory and relations. Visit byju’s to learn the statement of the transitive property, transitive property of equality and. Transitive relations are binary relations in set theory that are defined on a.
Transitive Verb Definition, Types of Transitive Verbs with Useful
It states that if two values are equal, and either of those two values. What is the transitive property in maths? Visit byju’s to learn the statement of the transitive property, transitive property of equality and. The transitive property is also known as the transitive property of equality. A transitive relation is a fundamental concept in mathematics, specifically in the.
DefinitionEquation ConceptsSymmetric Property of Equality Media4Math
What is the transitive property in maths? It states that if two values are equal, and either of those two values. The transitive property is also known as the transitive property of equality. Transitive relations are binary relations in set theory that are defined on a set a such that if a is related to b and b is related.
Transitive Relations Are Binary Relations In Set Theory That Are Defined On A Set A Such That If A Is Related To B And B Is Related To C, Then Element A.
It states that if two values are equal, and either of those two values. What is the transitive property in maths? The transitive property is also known as the transitive property of equality. A transitive relation is a fundamental concept in mathematics, specifically in the field of set theory and relations.