What Makes A Vector Field Conservative
What Makes A Vector Field Conservative - Explain how to find a potential function for a conservative vector field. We examine the fundamental theorem for line integrals, which is a useful generalization of the fundamental theorem of calculus to line integrals. How to determine if a vector field is conservative; In this section we will take a more detailed look at conservative vector fields than we’ve done in previous sections. The gradient theorem for line integrals; Use the fundamental theorem for line integrals to evaluate a line. The vector field \(\vecs{f} \) is said to be conservative if there exists a function \(\varphi\) such that \(\vecs{f} =.
In this section we will take a more detailed look at conservative vector fields than we’ve done in previous sections. The vector field \(\vecs{f} \) is said to be conservative if there exists a function \(\varphi\) such that \(\vecs{f} =. How to determine if a vector field is conservative; Explain how to find a potential function for a conservative vector field. The gradient theorem for line integrals; Use the fundamental theorem for line integrals to evaluate a line. We examine the fundamental theorem for line integrals, which is a useful generalization of the fundamental theorem of calculus to line integrals.
How to determine if a vector field is conservative; We examine the fundamental theorem for line integrals, which is a useful generalization of the fundamental theorem of calculus to line integrals. The gradient theorem for line integrals; In this section we will take a more detailed look at conservative vector fields than we’ve done in previous sections. Use the fundamental theorem for line integrals to evaluate a line. Explain how to find a potential function for a conservative vector field. The vector field \(\vecs{f} \) is said to be conservative if there exists a function \(\varphi\) such that \(\vecs{f} =.
Curl and Showing a Vector Field is Conservative on R_3 YouTube
The vector field \(\vecs{f} \) is said to be conservative if there exists a function \(\varphi\) such that \(\vecs{f} =. In this section we will take a more detailed look at conservative vector fields than we’ve done in previous sections. Use the fundamental theorem for line integrals to evaluate a line. We examine the fundamental theorem for line integrals, which.
What is a Conservative Vector Field? Wait, What is a Vector Field
How to determine if a vector field is conservative; In this section we will take a more detailed look at conservative vector fields than we’ve done in previous sections. The gradient theorem for line integrals; Explain how to find a potential function for a conservative vector field. Use the fundamental theorem for line integrals to evaluate a line.
We examine the fundamental theorem for line integrals, which is a useful generalization of the fundamental theorem of calculus to line integrals. Use the fundamental theorem for line integrals to evaluate a line. The gradient theorem for line integrals; Explain how to find a potential function for a conservative vector field. The vector field \(\vecs{f} \) is said to be.
potential function of a conservative vector field Vector Calculus
The gradient theorem for line integrals; We examine the fundamental theorem for line integrals, which is a useful generalization of the fundamental theorem of calculus to line integrals. How to determine if a vector field is conservative; Use the fundamental theorem for line integrals to evaluate a line. In this section we will take a more detailed look at conservative.
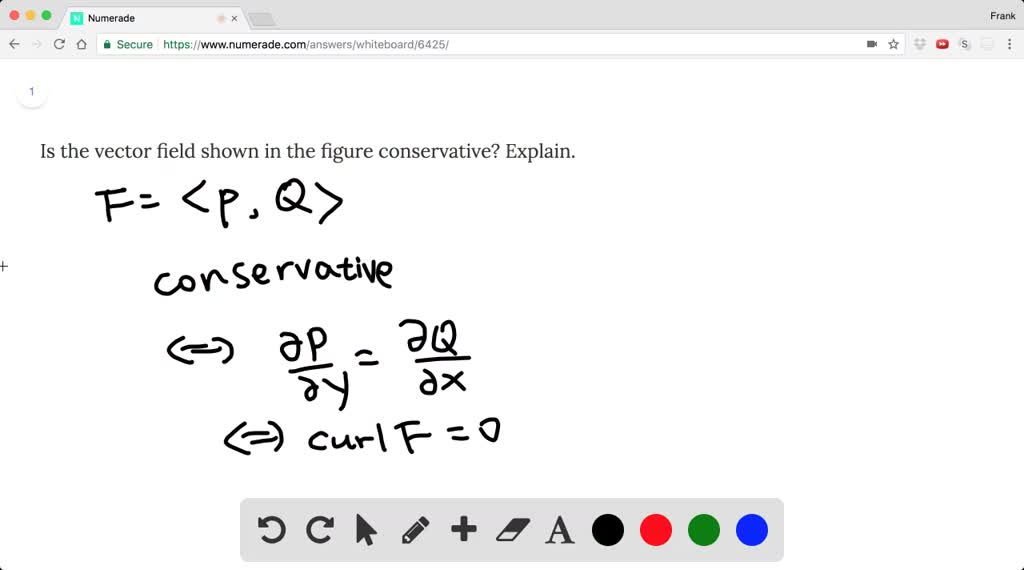
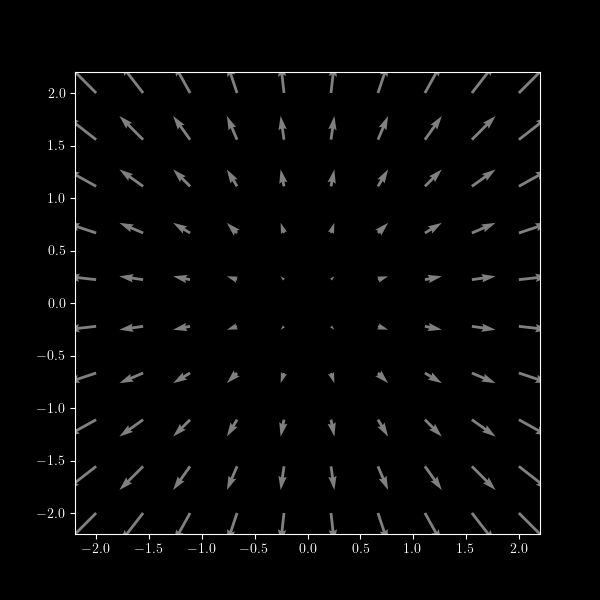
Is the vector field conservative? Explain. (GRAPH…
The vector field \(\vecs{f} \) is said to be conservative if there exists a function \(\varphi\) such that \(\vecs{f} =. In this section we will take a more detailed look at conservative vector fields than we’ve done in previous sections. Use the fundamental theorem for line integrals to evaluate a line. How to determine if a vector field is conservative;.
Determinación de la función potencial de un campo vectorial conservador
We examine the fundamental theorem for line integrals, which is a useful generalization of the fundamental theorem of calculus to line integrals. How to determine if a vector field is conservative; The gradient theorem for line integrals; Use the fundamental theorem for line integrals to evaluate a line. Explain how to find a potential function for a conservative vector field.
APMA E2000 Conservative Vector Fields & FTLI
Use the fundamental theorem for line integrals to evaluate a line. We examine the fundamental theorem for line integrals, which is a useful generalization of the fundamental theorem of calculus to line integrals. In this section we will take a more detailed look at conservative vector fields than we’ve done in previous sections. Explain how to find a potential function.
Conservative vector field Alchetron, the free social encyclopedia
How to determine if a vector field is conservative; The vector field \(\vecs{f} \) is said to be conservative if there exists a function \(\varphi\) such that \(\vecs{f} =. In this section we will take a more detailed look at conservative vector fields than we’ve done in previous sections. The gradient theorem for line integrals; We examine the fundamental theorem.
Bln The Natural Blog
Use the fundamental theorem for line integrals to evaluate a line. We examine the fundamental theorem for line integrals, which is a useful generalization of the fundamental theorem of calculus to line integrals. The vector field \(\vecs{f} \) is said to be conservative if there exists a function \(\varphi\) such that \(\vecs{f} =. In this section we will take a.
Conservative Vector Fields YouTube
We examine the fundamental theorem for line integrals, which is a useful generalization of the fundamental theorem of calculus to line integrals. Use the fundamental theorem for line integrals to evaluate a line. How to determine if a vector field is conservative; Explain how to find a potential function for a conservative vector field. In this section we will take.
In This Section We Will Take A More Detailed Look At Conservative Vector Fields Than We’ve Done In Previous Sections.
Explain how to find a potential function for a conservative vector field. Use the fundamental theorem for line integrals to evaluate a line. We examine the fundamental theorem for line integrals, which is a useful generalization of the fundamental theorem of calculus to line integrals. The gradient theorem for line integrals;
How To Determine If A Vector Field Is Conservative;
The vector field \(\vecs{f} \) is said to be conservative if there exists a function \(\varphi\) such that \(\vecs{f} =.